2 Answers

Cypherfunc · Answer 1 · 2020-11-21T16:36:04+0000

For this case we have:

Let k> 0:

To graph
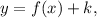y = f (x) + k, the graph k units is moved up.

To graph
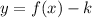y = f (x) -k , the graph moves k units down.

Let h> 0:

To graph
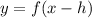y = f (x-h) , the graph moves h units to the right.

To graph
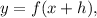y = f (x + h), the graph moves h units to the left.

So, we have:

$y = f (x) = \sqrt [3] {x}$

Shifted 1 unit down and 4 to the left means:

$k = 1\\h = 4$

$y = f (x) = \sqrt [3] {x + 4} -1$

Answer:

Option D

Please log in or register to add a comment.