Answer:
Yes, because the sum of all of the probabilities is 1.
Explanation:
The given probability equation is:
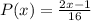 , where x belongs to {1,2,3,4}.
, where x belongs to {1,2,3,4}.
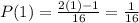
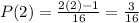
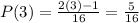
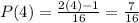
Let us sum all the probabilities to obtain;
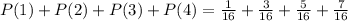
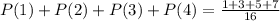
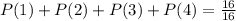
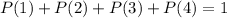
Since the sum of all the probabilities is 1, it is a probability distribution