Question

An Easter basket contains eggs of three different colors. Find the total number of eggs in the basket if 2 7 of all eggs are green, 1 4 of all are blue and the rest 26 eggs are red.

Answer 1

Answer: There are total 56 eggs in the basket.

Step-by-step explanation: Given that an Easter basket contains eggs of three different colors, out of which
`(2)/(7)` are green,
`(1)/(4)` are blue and rest 26 eggs are red.

We are to find the total number of eggs in the basket.

Let x be the total number of eggs in the Easter basket.

Then, according to the given information, we have

`(2)/(7)* x+(1)/(4)* x+26=x\\\\\\\Rightarrow (8x+7x)/(28)+26=x\\\\\\\Rightarrow (15x)/(28)+26=x\\\\\\\Rightarrow x-(15x)/(28)=26\\\\\\\Rightarrow (28x-15x)/(28)=26\\\\\\\Rightarrow (13x)/(28)=26\\\\\Rightarrow x=(26* 28)/(13)\\\\\Rightarrow x=56.`

Thus, there are total 56 eggs in the basket.

Answer 2

Total number of eggs in basket = 56

Explanation:

Let z be the total number of eggs

Green eggs =
`(2)/(7)` × z ........1

Blue eggs =
`(1)/(4)` × z .......2

Rest of eggs = 26 ........3

The addition of 1, 2 and 3 is equal to z

`(2)/(7)` × z +
`(1)/(4)` + 26 = z

`(8z + 7 z)/(28)` + 26 = z ∵GCF for 4 and 7 is 28

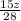`(15z)/(28)` + 26 = z

15z + (26 × 28) = 28z ∵ multiply both sides by 28

15z + 728 = 28z

28z - 15z = 728 .......... rearranging above equation

13z = 728 ⇒ z =
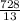`(728)/(13)` ⇒ z = 56

Total number of eggs = z = 56