Question

Design a new cereal box that will hold the same amount of cereal but reduce manufacturing costs. Prove that your new design holds the same amount but can be manufactured more cheaply.

*My box costs $11.6

So I have a rectangular prism thats
8 in-length
1 in-width
12 in-height
the volume is 96 in. and the surface area is 232
*If you could show your work that would be great. *

Answer 1

Step-by-step explanation:

Did you ever figure it out? I need help with this as well.

Answer 2

The proposed new cereal box design has dimensions of 6 inches in length, 2 inches in width, and 8 inches in height. This design maintains the original cereal volume of 96 cubic inches while reducing the surface area to 104 square inches.

Step-by-step explanation:

The formula for the surface area
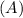`(\(A\))` of a rectangular prism is given by:

`\[ A = 2lw + 2lh + 2wh \]`

For the original box with dimensions 8 inches in length
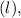`(\(l\)),` 1 inch in width
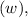`(\(w\)),` and 12 inches in height
`(\(h\))`:

`\[ A_{\text{original}} = 2(8 * 1) + 2(8 * 12) + 2(1 * 12) = 232 \]`

For the proposed new design with dimensions 6 inches in length, 2 inches in width, and 8 inches in height:

`\[ A_{\text{new}} = 2(6 * 2) + 2(6 * 8) + 2(2 * 8) = 104 \]`

Comparing the surface areas, the new design significantly reduces it from 232 square inches to 104 square inches. This reduction in surface area indicates that the proposed design will require less material for manufacturing.

By maintaining the original cereal volume of 96 cubic inches while decreasing the surface area, the proposed cereal box design is more cost-effective to manufacture. The optimization of dimensions ensures that the same cereal quantity can be accommodated with a reduction in manufacturing costs.