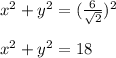Answer:
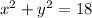
Explanation:
The standard equation of a circumference has the following formula.
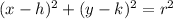
Where the point (h, k) is the center of the circle and r is the radius.
If in this case we know that the circle has center at point (0,0), then its equation will have the following form

The radius of the circumference will be the distance from the center of the circumference to the point where the circumference is tangent to the line
The radio is:
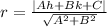
In this case, the line is
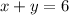
And the center of the circumference is (0, 0)
So
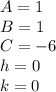
The radio is:
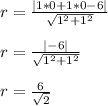
Finally the equation of the circumference is: