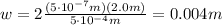Question

A 0.50 mm-wide slit is illuminated by light of wavelength 500 nm.What is the width of the central maximum on a screen 2.0m behind the slit?

Answer 1

0.004 m

Step-by-step explanation:

For light passing through a single slit, the position of the nth-minimum in the diffraction pattern is given by

`y=(n\lambda D)/(d)`

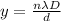
where

`\lambda` is the wavelength

D is the distance of the screen from the slit

d is the width of the slit

Therefore, the width of the central maximum is equal to twice the value of y for n=1 (first minimum):

`w=2(\lambda D)/(d)`

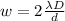
where we have

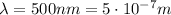`\lambda=500 nm = 5\cdot 10^(-7)m` is the wavelength

D = 2.0 m is the distance of the screen

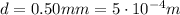`d=0.50 mm=5\cdot 10^(-4)m` is the width of the slit

Substituting, we find

`w=2((5\cdot 10^(-7) m)(2.0 m))/(5\cdot 10^(-4) m)=0.004 m`