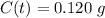Answer: Option E
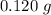
Explanation:
The exponential decay formula is:
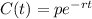
Where p is the initial amount of Ytterbium
r is the rate of decrease
C(t) is the amount of Ytterbium in grams as a function of time
t is the time in days
In this problem:
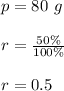
t is the time in units of 4.2 days
Then
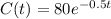
We want to calculate the amount of Ytterbium in 54.6 days.
Then as t is the time in units of 4.2 days
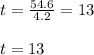
Finally