Answer:
UVA:
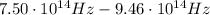
UVB:
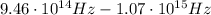
Step-by-step explanation:
The formula to calculate the frequency of each electromagnetic wave is:
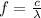
where
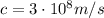 is the speed of light
is the speed of light
 is the wavelength
is the wavelength
For UVA:
- The minimum wavelength is
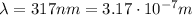
so the frequency is

- The maximum wavelength is
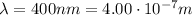
so the frequency is
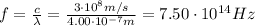
For UVB:
- The minimum wavelength is
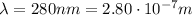
so the frequency is
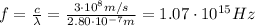
- The maximum wavelength is
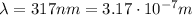
so the frequency is
