Answer:
To the nearest tenth, the height of the balloon is 2.9 miles
Explanation:
The nearer point takes the greater angle of elevation.
The diagram is shown in the attachment.
The height of the balloon above the ground is c unit.
From triangle ABD,
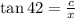
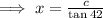 ...eqn1
...eqn1
From triangle ABC,

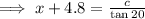
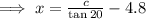 ..eqn2
..eqn2
We equate both equations and solve for c.

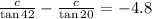
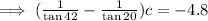
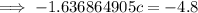

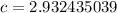
To the nearest tenth, the height of the balloon is 2.9 miles