Question

For the exponential equation
`y = 3.4(0.66)^x` is it a growth or decay function and what is the y-intercept and the rate of growth or decay?

A) Growth, y- intercept = 1, rate is 66%

B) Decay, y- intercept = 3.4, rate is 34%

C) Decay, y- intercept = 3.4, rate is 66%

D) Growth, y- intercept = 3.4, rate is 34%

Answer 1

Option: B is the correct answer.

B) Decay, y- intercept = 3.4, rate is 34%

Explanation:

We are given an exponential equation as follows:

`y=3.4(0.66)^x`

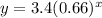
We know that any exponential function of the type:

`y=ab^x`

is growth function if: b>1

and is a decay function if 0< b< 1

Hence, here we have: b=0.66<1

Hence, the function is a decay function.

Also, we know that the y-intercept of a function is the point where x=0

Hence, we take x=0

we have:
`y=3.4* (0.66)^0\\\\\\y=3.4`

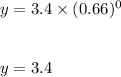
Hence, the y-intercept is: 3.4

Also, the rate of a function is denoted by 'r'

where b=1-r

⇒ 0.66=1-r

⇒ r=1-0.66

⇒ r=0.34

Hence, the rate is: 34%

option: B is the correct answer.