Answer:
 or
or
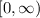
Explanation:
By definition the function
 has a domain of
has a domain of
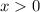 .
.
Since the function is not defined for the values of x negative or equal to zero.
The range of this function is all the real numbers.
Since
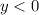 when
when
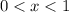 and
and
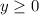 when
when

In this case we have the function
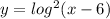
Therefore since the log function is squared then its range is now
