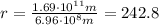Question

The Schwarzschild radius is the distance from an object at which the escape velocity is equal to the speed of light. A black hole is an object that is smaller than its Schwarzschild radius, so not even light itself can escape a black hole. The Schwarzschild radius ???? depends on the mass ???? of the black hole according to the equation ????=2????????????2 where ???? is the gravitational constant and ???? is the speed of light. Consider a black hole with a mass of 5.7×107M⊙. Use the given equation to find the Schwarzschild radius for this black hole. Schwarzschild radius: m What is this radius in units of the solar radius

Answer 1

1.
`1.69\cdot 10^(11) m`

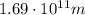
The Schwarzschild radius of an object of mass M is given by:

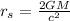`r_s = (2GM)/(c^2)` (1)

where

G is the gravitational constant

M is the mass of the object

c is the speed of light

The black hole in the problem has a mass of

`M=5.7\cdot 10^7 M_s`

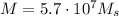
where

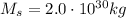`M_s = 2.0\cdot 10^(30) kg` is the solar mass. Substituting,

`M=(5.7\cdot 10^7)(2\cdot 10^(30)kg)=1.14\cdot 10^(38) kg`

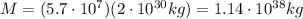
and substituting into eq.(1), we find the Schwarzschild radius of this black hole:

`r_s = (2(6.67\cdot 10^(-11))(1.14\cdot 10^(38) kg))/((3\cdot 10^8 m/s)^2)=1.69\cdot 10^(11) m`

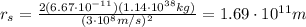
2) 242.8 solar radii

We are asked to find the radius of the black hole in units of the solar radius.

The solar radius is

`r_S = 6.96\cdot 10^5 km = 6.96\cdot 10^8 m`

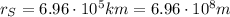
Therefore, the Schwarzschild radius of the black hole in solar radius units is

`r=(1.69\cdot 10^(11) m)/(6.96\cdot 10^8 m)=242.8`