Answer:
Here's a possible example:
Explanation:
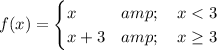
Each piece is linear, so the pieces are continuous by themselves.
We need consider only the point at which the pieces meet (x = 3).
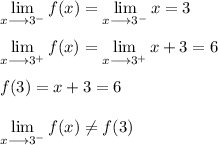
The left-hand limit does not equal ƒ(x), so there is a jump discontinuity at x =3.