Answer:

Equation of the line

Explanation:
To find the slope we need two points,
 and
and
 In this case we have the points
In this case we have the points
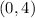 and
and
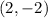
 (1)
(1)
We replace the points in the equation (1)
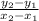
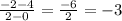
We know the equation of the line:
 (2)
(2)
To find b we replace the slope, x and y with one of the points in the equation (2)
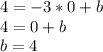
We substitute m and b in the general equation of the line
