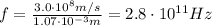Question

The Cosmic Background Radiation Outer space is filled with a sea of photons, created in the early moments of the universe. The frequency distribution of this "cosmic background radiation" matches that of a blackbody at a temperature near 2.7K.What is the peak frequency of this radiation in Hz?What is the wavelength that corresponds to the peak frequency in mm?

Answer 1

A) Peak wavelength: 1.07 mm

The peak wavelength of the Cosmic Background Radiation can be found by using Wien's displacement law:

`\lambda = (b)/(T)`

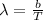
where

`\lambda` is the peak wavelength

`b=2.898\cdot 10^(-3)m\cdot K` is Wien's displacement constant

T is the absolute temperature

For the Cosmic Background Radiation,

T = 2.7 K

So the peak wavelength is

`\lambda = (2.898\cdot 10^(-3)m\cdot K)/(2.7 K)=1.07\cdot 10^(-3) m=1.07 mm`

B) Peak frequency:
`2.8\cdot 10^(11)Hz`

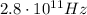
The peak frequency can be found by using the relationship:

`f=(c)/(\lambda)`

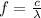
where

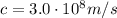`c=3.0\cdot 10^8 m/s` is the speed of light

`\lambda` is the peak wavelength

Substituting numbers, we find

`f=(3.0\cdot 10^8 m/s)/(1.07\cdot 10^(-3) m)=2.8\cdot 10^(11)Hz`