Question

Suppose that the sun shrank in size but that its mass remained the same. What would happen to the orbit of the earth?

Answer 1

Nothing

Step-by-step explanation:

The radius of the orbit of the Earth does not depend on the radius of the sun.

In fact, the gravitational attraction between the Earth and the Sun provides the centripetal force that keeps the Earth in orbit:

`G(Mm)/(r^2) = m(v^2)/(r)`

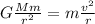
where

G is the gravitational constant

M is the mass of the sun

m is the mass of the Earth

r is the radius of the orbit of the Earth

v is the orbital speed of the earth

Re-arranging the equation for r:

`r=(GM)/(v^2)`

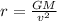
Also,

`v=\omega r`

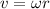
where
`\omega` is the angular velocity of the Earth's orbit. So we can rewrite the equation as

`r=(GM)/(\omega^2 r^2)\\r^3 = (GM)/(\omega^2)`

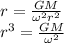
As we see, the radius of the orbit of the Earth, r, does not depend on the mass of the Sun, so if the sun shrank in size, the orbit remains the same.