Question

What are the answers and why?

Answer 1

`\boxed{\text{(v) and (viii)}}`

Explanation:

The three step test for continuity states that a function ƒ(x) is continuous at a point x = a if three conditions are satisfied:

• f(a) is defined.
• The limit of ƒ(x) as x approaches a exists.
• The limit of ƒ(x) as x approaches a is equal to f(a).

(i) Left-hand limit = right-hand limit.

Pass. The limit from either side is 8.

(ii) Left-hand limit = limit.

Pass. If the limits from either direction exist, the limit exists.

(iii) Limit as x ⟶ ∞ is not part of the three-step test.

(iv) Limit as x ⟶ 1 exists. Pass.

(v) f(1) is defined.

FAIL. f(1) is not defined.

(vi) Limit as x ⟶ ∞ is not part of the three-step test.

(vii) Passing the three-step test is not a step in the test.

(viii) The limit as x ⟶ 1 does not equal f(1).

FAIL. f(1) is undefined.

The steps in the three-step test for which the function fails are
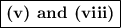`\boxed{\textbf{(v) and (viii)}}`.