Answer:

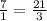
Explanation:
Verify each case
case 1)

Multiply in cross and then compare both sides
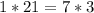
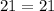 ----> is true
----> is true
therefore
Is a valid proportion
case 2)
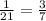
Multiply in cross and then compare both sides
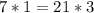
 ----> is not true
----> is not true
therefore
Is not a valid proportion
case 3)
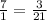
Multiply in cross and then compare both sides
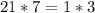
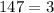 ----> is not true
----> is not true
therefore
Is not a valid proportion
case 4)
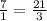
Multiply in cross and then compare both sides
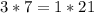
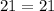 ----> is true
----> is true
therefore
Is a valid proportion
case 5)
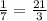
Multiply in cross and then compare both sides
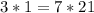
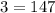 ----> is not true
----> is not true
therefore
Is not a valid proportion