For this case, we must expand the series for each value of "n". That is to say:
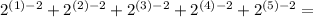
We have that equal signs are added and the same sign is placed, while different signs are subtracted and the sign of the major is placed:
2 ^ {1-2} + 2 ^ {2-2} + 2 ^ {3-2} + 2 ^ {4-2} + 2 ^ {5-2} =

By definition of power properties we have to:
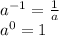
So:

We find the common denominator:
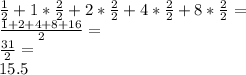
Answer:
Option B