Help with this homework pls

Question

asked Jul 17, 2022 178k views

1 Answer

Kreo · Answer 1 · 2022-07-22T01:58:37+0000

Answer:

We conclude that:

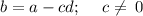
$b=a-cd;\quad \:c\\e \:0$

Hence, option A is true.

Explanation:

Given the expression

(a-b)/(c)=d

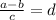
Solving for b

(a-b)/(c)=d

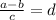
Multiply both sides by c

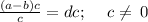
$(\left(a-b\right)c)/(c)=dc;\quad \:c\\e \:0$

simplify

$a-b=dc;\quad \:c\\e \:0$

Subtract a from both sides

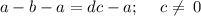
$a-b-a=dc-a;\quad \:c\\e \:0$

Simplify

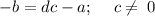
$-b=dc-a;\quad \:c\\e \:0$

Divide both sides by -1; c≠0

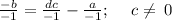
$(-b)/(-1)=(dc)/(-1)-(a)/(-1);\quad \:c\\e \:0$

simplify

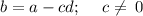
$b=a-cd;\quad \:c\\e \:0$

Therefore, we conclude that:

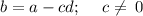
$b=a-cd;\quad \:c\\e \:0$

Hence, option A is true.