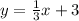Question

A line passes through the origin and has a slope of -3 what is the equation of the line that is perpendicular to the first line and passes through the point (3,4)?

Answer 1

`y= (1)/(3) x + 3`

EXPLANATION

We want to find the equation of a line which is perpendicular to another line with slope -3 and passes through (3,4).

Our line of interest is has a slope that is the negative reciprocal of -3

`m = - (1)/( - 3) = (1)/(3)`

The equation is given by

`y-y_1=m(x-x_1)`

We substitute the point and slope to get:

`y-4= (1)/(3) (x-3)`

Expand

`y-4= (1)/(3) x-1`

`y= (1)/(3) x-1 + 4`

`y= (1)/(3) x + 3`

Answer 2

Answer:
`y=(1)/(3)x+3`

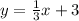
Explanation:

The equation of the line is Slope-intercept form is:

`y=mx+b`

Where "m" is the slope and "b" the y-intercept.

The slopes of perpendicular lines are negative reciprocal.

Then, if the slope of the first line is -3, the slope of the other line must be:

`m=(1)/(3)`

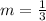
Substitute the point (3,4) into the equation and solve for b:

`4=(1)/(3)(3)+b\\ 4-1=b\\b=3`

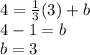
Then the equation of this line is:

`y=(1)/(3)x+3`