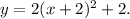Answer:
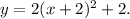
Explanation:
For a quadratic equation written in the general form
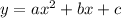 ,
,
the x coordinate of its vertex is:
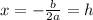
Then this equation written in the form of vertex is:

Where the point (h, k) is the vertex of the parabola.
In this case we have the equation

Then the x coordinate of its vertex is:
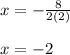
Therefore the y coordinate of its vertex is:
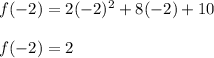
The vertice is (-2, 2)
Then
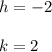 .
.
This equation written in the form of vertex is