Answer:
The correct option is 2.
Explanation:
The given system of equations is
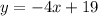 ..... (1)
..... (1)
 ..... (2)
..... (2)
The slope intercept form of a line is
 .... (3)
.... (3)
where, m is slope and b is y-intercept.
From (1) and (3), we get

The slope of first line is -4 and the y-intercept is 19. It means it is a decreasing line and intersect the y-axis at (0,19).
From (2) and (3), we get

The slope of first line is 2 and the y-intercept is 1. It means it is an increasing line and intersect the y-axis at (0,1).
Put y=0, to find the x-intercepts.
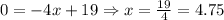

Therefore the x-intercept of first line is 4.75 and the x-intercept of the second line is -0.5.
Only the second graph satisfy all the above condition.
One solving the given equation we get
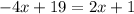
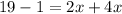

Divide both sides by 6.
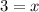
Put this value in equation (1).
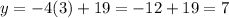
Therefore the solution of the given system of equation is (3,7).
Hence the correct option is 2.