Question

Identify the center and the radius of each.

x^+y^+26x-22y+281=0

Answer 1

Center (-13,11) , radius 3

Explanation:

The given circle has equation

`x^2+y^2+26x-22y+281=0`

We compare this to the general equation.

`x^2+y^2+2ax+2by+c=0`

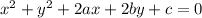
where (-a,-b) is the center.

This implies that;

2a=26

`\Rightarrow a=13`

2b=-22

b=-11

The center is therefore (-13,11)

The radius is given by

`r=√(a^2+b^2-c)`

`r=√(13^2+(-11)^2-281)`

`r=√(9)`

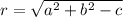
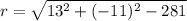
The radius is 3