Question

A television manufacturer decides to increase its production by 25% per month to meet increasing customer demand. The company currently produces 2,000 television sets a month.

Which of the following graphs shows the total number of television sets, y, manufactured by the company in x months?

Answer 1

Answer: the last ones

Step-by-step explanation: I put it and got it right:)

Answer 2

The last graph

Explanation:

The problem presented here is similar to a compound interest problem since we have an initial value, a growth constant and the aspect of time.

We can consider the number of television sets currently produced by the company to be our Principal amount;

P = 2000

The rate of increase in production per month can be considered as our interest rate earned;

r = 25% = 0.25

The total number of television sets y will be our Accumulated amount;

A = y

The duration x becomes our time n.

The compound interest formula is given as;

`A=P(1+r)^(n)`

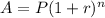
We simply substitute the given information into the formula;

`y=2000(1.25)^(x)`

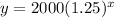
This is an exponential growth function since the base of the exponent x is greater than 1.

A graph of the function will be an exponential curve passing through ( 0, 2000) since 2000 is our initial value