Question

How do you Graph y-3=1/3(x+1)

Answer 1

You can find the point of intersection of the line with the y-axis and the the point of intersection of the line with the x-axis. See the graph attached.

Explanation:

Find the intersection with the y-axis. Substitute
`x=0` into the equation and solve for y:

`y-3=(1)/(3)(x+1)\\\\y-3=(1)/(3)(0+1)\\\\y-3=(1)/(3)(1)\\y=(1)/(3)+3\\\\y=(10)/(3)`

`y`≈3.33

Find the intersection with the x-axis. Substitute
`y=0` into the equation and solve for x:

`y-3=(1)/(3)(x+1)`

`0-3=(1)/(3)(x+1)\\(-3)(3)=x+1\\-9-1=x\\x=-10`

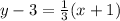
Now, you know that the line passes through the point (0,3.33) and (-10,0). Now you can graph the function. (Observe the graph attached)