Question

27) A man on the third floor of a building shouts down to a person on the street. If the man is 25 feet up and the distance between the person on the street and the man in the building is 50 feet, what is the angle of elevation (in degrees) between the person on the street and the person in the building?

A) 15°
B) 30°
C) 45°
D) 60°

Answer 1

Its B

Explanation:

I just did the test.

Answer 2

Option B) 30°

Explanation:

Given : A man on the third floor of a building shouts down to a person on the street. If the man is 25 feet up and the distance between the person on the street and the man in the building is 50 feet.

To find : What is the angle of elevation (in degrees) between the person on the street and the person in the building?

Solution :

According to question, a rough diagram is framed which shows the position of man on street and man on building.

Refer the attached figure below.

A man on the third floor of a building is 25 feet up i.e. AB=25 feet.

The distance between the person on the street and the man in the building is 50 feet i.e. BC=50 feet.

We have to find the angle of elevation i.e. ∠C.

It form a right angle triangle,

Applying sin property of trigonometric,

`\sin \theta=\frac{\text{Perpendicular}}{\text{Hypotenuse}}`

`\sin \theta=(AB)/(BC)`

`\sin \theta=(25)/(50)`

`\sin \theta=(1)/(2)`

`\sin \theta=\sin 30^\circ`

`\theta=30^\circ`

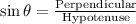
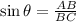
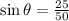
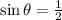
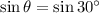
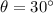
Therefore, Option B is correct.

The angle of elevation is 30°.