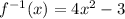Question

Find the inverse of the given function.

Answer 1

`f(x)^(-1)= 4x^(2) -3` .

Explanation:

Given :
`f(x) =-(1)/(2)√(x+3)`.

To find : Find the inverse of the given function.

Solution : We have given

`f(x) =-(1)/(2)√(x+3)`.

Step 1: take f(x) = y

`y =-(1)/(2)√(x+3)`.

Step 2 : Inter change y and x.

`x =-(1)/(2)√(y+3)`.

Step 3 : Solve for y

Taking square both sides

`x^(2) = (1)/(4)(y+3)`.

On multiply both sides by 4.

`4x^(2) = (y+3)`.

On subtraction both sides by 3.

`4x^(2) -3 = y`.

Here,
`f(x)^(-1)= y` is inverse of f(x)

`f(x)^(-1)= 4x^(2) -3` .

Therefore,
`f(x)^(-1)= 4x^(2) -3` .

Answer 2

For this case we must find the inverse of the following function:

`f (x) = - \frac {1} {2} \sqrt {x + 3}`

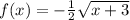
We follow the steps below:

Replace f(x) with y:

`y = -\frac {1} {2} \sqrt {x + 3}`

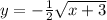
We exchange the variables:

`x = - \frac {1} {2} \sqrt {y + 3}`

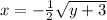
We solve for "y":

`- \frac {1} {2} \sqrt {y + 3} = x`

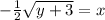
Multiply by -2 on both sides of the equation:

`\sqrt {y + 3} = - 2x`

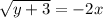
We raise both sides of the equation to the square to eliminate the radical:

`(\sqrt {y + 3}) ^ 2 = (- 2x) ^ 2\\y + 3 = 4x ^ 2`

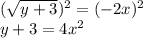
We subtract 3 from both sides of the equation:

`y = 4x ^ 2-3`

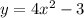
We change y by f ^ {- 1} (x):

`f ^ {- 1} (x) = 4x ^ 2-3`

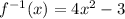
Answer:
`f ^ {- 1} (x) = 4x ^ 2-3`