Question

Which conic section does the equation below describe?


`((x-9)^2)/(4) + ((y+2)^2)/(25) =1`

A. Hyperbola
B. Circle
C. Ellipse
D. Parabola

Answer 1

C

Explanation:

Answer 2

Answer: Option C. Ellipse

Explanation:

The general equation of an ellipse has the following form:

`((x-h)^2)/(a^2) + ((y-k)^2)/(b^2) =1`

Where the point (h, k) is the center of the ellipse

In this case we have the equation

`((x-9)^2)/(4) + ((y+2)^2)/(25) =1`

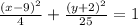
Note that its shape matches that of a ellipse with center (9, -2)

`a=√(4) \\a=2\\\\\\b=√(25)\\b=5`

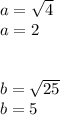
Therefore the answer is the obtion C