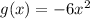Question

If f(x) = x^2 is vertically stretched by a factor of 6 to g(x) and reflected over the x-axis, what is the equation for g(x)?

A. g(x) = (-6x)^2
B. g(x) = -6x^2
C. g(x) = x^2-6
D. g(x) = -x^2+6

Answer 1

Option B

`g(x) = -6x^2`

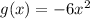
Explanation:

If the graph of the function
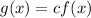`g(x)=cf(x)` represents the transformations made to the graph of
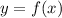`y= f(x)` then, by definition:

If
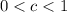`0 <c <1` then the graph is compressed vertically by a factor c.

If
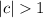`|c| > 1` then the graph is stretched vertically by a factor c

If
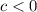`c <0` then the graph is reflected on the x axis.

In this problem we have the function
`f(x)=x^2`

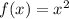
We now that this function is vertically stretched by a factor of 6 to g(x) and reflected over the x-axis

Then
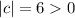`|c| =6 >0` and
`c=-6<0`

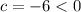
Therefore the graph of
`g(x)` is
`g(x) = -6f(x)`

`g(x) = -6x^2`