Question

Please help I need it

Answer 1

There’s an app called Socratic it will help u with the steps

Answer 2

Question 1:

For this case we have to simplify the following expression:

`\sqrt {54n ^ 7}`

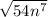
We can rewrite the 54 as:

`54 = 9 * 6 = 3 ^ 2 * 6`

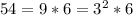
In addition, we have to:

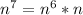`n ^ 7 = n ^ 6 * n` (According to the multiplication of powers of the same base)

Also, by definition of properties of powers and roots we have to:

\sqrt [n] {a ^ m} = a ^ (\frac {m} {n})

Then, we can rewrite the expression as:

`\sqrt {3 ^ 2 * n ^ 6 * 6 * n} =\\3n ^ 3 \sqrt {6n}`

Answer:

Option C

Question 2:

For this case we have by definition, that a perfect square is the result of multiplying a number by itself. Also, the perfect squares are the numbers that have exact square roots.

So:

2 and 10 are not perfect squares.

Answer:

Option A and D

Question 3:

For this case we must simplify the following expression:

`\sqrt {75x ^ 2 * y ^ 4}`

We can rewrite the 75 as:
`75 = 25 * 3 = 5 ^ 2 * 3`

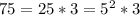
Also we have that by definition of properties of powers and roots that:

`\sqrt [n] {a ^ m} = a ^ (\frac {m} {n})`

So, we rewrite the expression:

`\sqrt {5 ^ 2 * x ^ 2 * y ^ 4 * 3} =\\5xy ^ 2 \sqrt {3}`

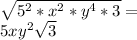
Answer:

Option B