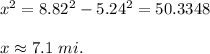Answer:
7.1 miles
Explanation:
Consider right triangle HospitalSchoolSupermarket. In this triangle:
- HospitalSchool = 14.7 mi;
- HospitalSupermaket = 11.76 mi;
- School Supermarket = 8.82 mi.
The shortest road from the main entrance of the supermarket to the highway that connects the school and hospital will be the height drawn from the point Supermarket to the hypotenuse HospitalSchool.
Let the length of this road be x mi and the distance from School to point A be y mi. Use twice the Pythagorean theorem for right triangles Supermarket SchoolA and SupermarketHospitalA:
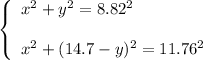
Subtract from the second equation the first one:

Thus,