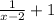Answer:
f(x) =
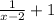
Explanation:
First we identify the Vertical Asymptote. Which is at x = 2
The function has a VA only at the point where the denominator gives 0, so because we know x = 2 is the VA, the denominator will be x - 2.
Then we identify the Horizontal Asymptote, which is the behavior of the function for very large 'x', or the horizontal line of the graph on the y - axis. Which is at y = 1. So our function will go to 1, as x grows incredibly large.
But since our denominator is x - 2, the top remains 1, but we should add a 1 to the function so it really has a HA at y = 1.
f(x) =