Question

8. What is the force of gravity on a dog in a space suit that's running around on the moon? The dog's body has a mass of 20 kilograms. Round to the nearest hundredth place. A. 196 N B. 1,176 N C. 2 N D. 32.67 N

Answer 1

Weight = (mass) x (acceleration of gravity)

Gravity on or near the moon's surface = 1.63 m/s^2

Dog's weight = (20 kg) x (1.63 m/s^2)

Weight = 32.6 Newtons (D)

Answer 2

D. around 32 N.

Given that:

• The mass of the moon is approximately
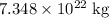  `7.348* 10^(22)\;\text{kg}`, and
• The (mean) radius of the moon is approximately
  `1.7371* 10^(6)\;\text{m}`.

Step-by-step explanation:

The dog is much smaller and lighter than the moon; it behaves like a point mass. Consider the equation for the size of gravity between a spherical mass and a point mass outside that spherical mass:

`\displaystyle F = (G\cdot M \cdot m)/(r^(2))`,

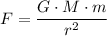where

• 
  `F` is the size of gravity,
• The gravitational constant
  `G \approx 6.67* 10^(-11)\;\text{kg}^(-1)\cdot \text{m}^(-1)\cdot \text{s}^(-2)`,
• 
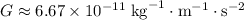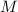  `M` is the mass of the sphere,
• 
  `m` is the size of the point mass, and
• 
  `r` is the separation between the point mass and the center of mass of the sphere.

The dog is at the surface of the moon. As a result, the
`r` shall be the same as the radius of the moon. Make sure all values are in SI units (kilograms and meters.) Apply the formula:

`\displaystyle \begin{aligned}F &= (G\cdot M \cdot m)/(r^(2)) \\ &= ((6.67* 10^(-11))*(7.348* 10^(22))* 20)/((1.7371* 10^(6))^(2))\\&= 32.48\;\text{N}\end{aligned}`.

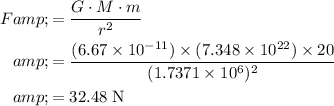This value may vary slightly depending on the position of the dog on the moon.