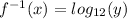Answer:
Explanation:
We have the following function
y = 12^x, and we need to find the inverse function.
To find the inverse function we should solve the equation for "x". To do so, first, we need to:
1. Take the logarithm in both sides of the equation:
lg_12 (y) = log _12 (12^x)
(Please read lg_12 as: "Logarithm with base 12")
From property of logarithm, we know that lg (a^b) = b*log(a)
Then:
lg_12 (y) = x*log _12 (12)
We also know that log _12 (12) = 1
Then:
x = log_12(y).
Then, the inverse of: y= 12^x is: