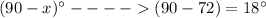Question

The complement of an angle is one-sixth the measure of the supplement of the angle. What is the measure of the complement angle?

A) 14°
B) 16°
C) 18°
D) 20°

Answer 1

Option C. 18

Explanation:

Let x be the given angle.

1

6

(180 − x) = (90 − x)

30 −

1

6

x = 90 − x

5

6

x = 60

x = 72°

The supplement angle to 72° is 108°.

The complement angle to 72° is 18°.

Answer 2

Option C.

The measure of the complement angle is
`18\°`

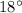
Explanation:

Let

x-----> the angle

we know that

The complement of an angle is equal to
`(90-x)\°`

The supplement of an angle is equal to
`(180-x)\°`

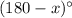
we have

The complement of an angle is one-sixth the measure of the supplement of the angle

`(90-x)\°=(1/6)(180-x)\°`

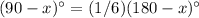
solve for x

`(540-6x)\°=(180-x)\°\\ (6x-x)=(540-180)\°\\ (5x)=(360)\°\\ x=72\°`

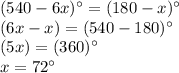
Find the measure of the complement angle

`(90-x)\° ----> (90-72)=18\°`