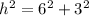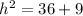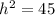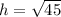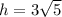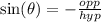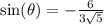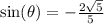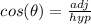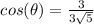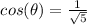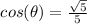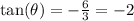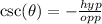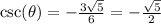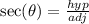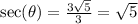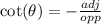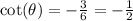ANSWER
See explanation
Step-by-step explanation
The point (3,-6) is the fourth quadrant.
In this quadrant only the cosine ratio and the secant ratio are positive.
The remaining four trigonometric ratios are negative.
The diagram is shown in the attachment.
We use the Pythagoras Theorem to find the hypotenuse, h.