Question

Find the surface area of the open top flower box shown

Answer 1

The surface area of box = 64 ft²

Explanation:

From the figure we can see that a cuboid with length = 10 ft, width = 2 ft and height = 2 ft

To find the surface area of cuboid

Surface area = 2(lb + bh + lh) - lh

= [2(10 * 2) + (2 * 2) + (10 * 2)] - (10 * 2)

= [2( 20 + 4 + 20)] - 20

= 2 * 44 - 20 = 84 - 20 = 64 ft²

The correct answer is surface area of box = 64 ft²

Answer 2

Answer:
`68ft^2`

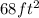
Explanation:

The formula for calculate the surface area of a rectangular prism is:

`SA=2lw+2lh+ 2wh`

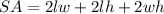
Where "l" is the lenght, "w" is the width and "h" is the height.

In this case, you know that the top of the flower box is opened, then, the formula changes to:

`SA=lw+2lh+2wh`

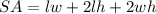
You can identify that the dimensions of the box are:

`l=10ft\\w=2ft\\h=2ft`

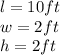
Then you must substitute these values into the formula
`SA=lw+2lh+2wh`.

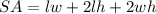Finally, you get that the surface area of the open-top flower box is:

`SA=lw+2lh+ 2wh\\SA=(10ft)(2ft)+(2)(10ft)(2ft)+(2)(2ft)(2ft)]\\SA=68ft^2`