Question

Kalaty bought 1/12 pounds of butter.How many ounces are 1/12 pounds of butter? Layla hatched 92 eggs.She used 43 and 1/5 of them how many eggs does she have left?

Answer 1

For this case we have that by definition:

1 pound is equivalent to 16 ounces.

We make a rule of three to determine the ounces of butter:

1lb ------------------------> 16onzas

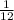`\frac {1} {12}`----------> x

Where "x" represents the ounces of butter

`x = \frac {\frac {1} {12} * 16} {1}\\x = \frac {16} {12}\\x = \frac {8} {6}\\x = \frac {4} {3}`

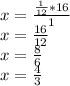
Thus, there are
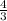`\frac {4} {3}`ounces of butter.

Now we must find the amount of eggs that remain:

`92- (43+ \frac {1} {5}) =\\92 - (\frac {43 * 5 + 1} {5}) =\\92 - (\frac {215 + 1} {5}) =\\92 - (\frac {216} {5}) =\\92-43.2 =\\48.8`

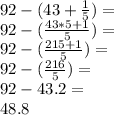
Round down. There are 48 eggs left.

Answer:

There are
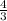`\frac {4} {3}` ounces of butter.

48 eggs left