Question

At a local company, the ages of all new employees hired during the last 10 years are normally distributed. The mean age is 32 years old, with a standard deviation of 10 years. Find the percent of new employees that are at least 25 years old. Round to the nearest percent.

Answer 1

P = 76%

Explanation:

We look for the percentage of employees who are at least 25 years old.

We know that:

μ = 32 years

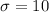`\sigma = 10` years

And we want to find

`P(X\geq25)`

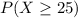
Then we find the z-score

`Z =(X - \mu)/(\sigma)`

So

`Z = -0.7`

Then

`P (X\geq25) = P((X- \mu)/(\sigma)\geq(25-32)/(10))\\\\\P (X\geq25) = P (Z\geq -0.7)`

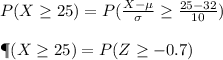
By symmetry of the distribution

`P(Z\geq -0.7)= 1-P(Z<-0.7)`

`P(Z\geq -0.7)= 1-0.242`

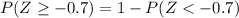
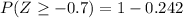
Looking in the normal standard tables

`P(Z\geq -0.7)= 0.758`

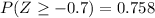
Finally P = 76%