Question

The graph shows which inequality? The vertex is (-1,3)

Answer 1

Answer: Last Option

`y> | x + 1 | +3`

Explanation:

First we must identify the function shown in the graph.

It is a function of absolute value whose vertex is in the point (-1, 3)

The absolute value parent function is:

`h(x) = | x |`. And it has its vertex in (0, 0)

The function shown in the graph is the function h(x) displaced 1 unit to the left and 3 units to the top.

Therefore the function shown in the graph
`f(x) = h (x + 1) +3`

`f (x) = | x + 1 | +3`.

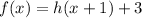
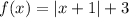Then, the region shaded in the graph are all the values of y that are above the graph of
`f (x) = | x + 1 | +3`

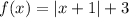
That is, the region is formed by all values where y is greater than
`f (x) = | x + 1 | +3`

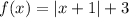
Then the inequality is:

`y> | x + 1 | +3`