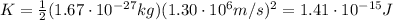A proton accelerates from rest in a uniform electric field of 635 N/C. At some time later, its speed is 1.30 × 106 m/s. What is the magnitude of its acceleration? The mass of a proton is 1.6726 × 10−27 - QAmmunity.org

A proton accelerates from rest in a uniform electric field of 635 N/C. At some time later, its speed is 1.30 × 106 m/s. What is the magnitude of its acceleration? The mass of a proton is 1.6726 × 10−27

asked Jun 27, 2020 86.5k views

1 Answer

1)
$6.11\cdot 10^(10) m/s^2$

The force experienced by the proton is

F=qE

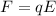
where

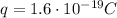$q=1.6\cdot 10^(-19)C$ is the proton charge

E=635 N/C is the strength of the electric field

Substituting into the equation,

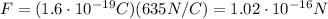
$F=(1.6\cdot 10^(-19) C)(635 N/C)=1.02\cdot 10^(-16)N$

The acceleration of the proton is given by Newton's second law:

a=(F)/(m)

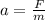
where

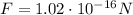$F=1.02\cdot 10^(-16)N$ is the force exerted on the proton

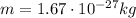$m=1.67\cdot 10^(-27) kg$ is the proton's mass

Substituting,

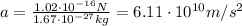
$a=(1.02\cdot 10^(-16)N)/(1.67\cdot 10^(-27)kg)=6.11\cdot 10^(10) m/s^2$

2)
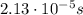
$2.13\cdot 10^(-5) s$

We can use the following equation:

a=(v-u)/(t)

where

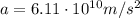$a=6.11\cdot 10^(10) m/s^2$ is the acceleration of the proton

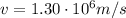$v=1.30\cdot 10^6 m/s$ is the final velocity

u = 0 is the initial velocity

t is the time

Solving the equation for t, we find

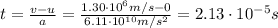
$t=(v-u)/(a)=(1.30\cdot 10^6 m/s -0)/(6.11\cdot 10^(10) m/s^2)=2.13\cdot 10^(-5) s$

3) 13.86 m

The distance travelled by the proton is given by the equation

d=ut + (1)/(2)at^2

where

u = 0 is the initial velocity

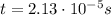$t=2.13\cdot 10^(-5) s$ s the time

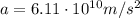$a=6.11\cdot 10^(10) m/s^2$ is the acceleration of the proton

Substituting,

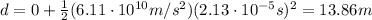
$d=0 + (1)/(2)(6.11\cdot 10^(10)m/s^2)(2.13\cdot 10^(-5) s)^2=13.86 m$

4)
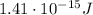
$1.41\cdot 10^(-15) J$

The final kinetic energy of the proton is given by

K=(1)/(2)mv^2

where we have

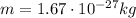$m=1.67\cdot 10^(-27) kg$ is the proton's mass

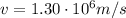$v=1.30\cdot 10^6 m/s$ is the final velocity

Substituting into the formula,

$K=(1)/(2)(1.67\cdot 10^(-27)kg)(1.30\cdot 10^6 m/s)^2=1.41\cdot 10^(-15) J$

Aleksandr Erokhin answered Jul 2, 2020

by Aleksandr Erokhin

4.9k points

Search QAmmunity.org