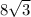Question

I have no idea what I’m doing.... help please!

Answer 1

Explanation:

In a 45°, 45°, 90° triangle, Both legs are equal and the hypotenuse is the leg lengths multiplied by the square root of 2.

• Given a triangle with a 45° and 90° angle, the other angle must also be 45°.

• In any of these triangles, the hypotenuse is
  `√(2)` times the side length. You can also find the side length by dividing the hypotenuse by
  `√(2)`.

• You can also use this to determine both the legs are the same length. This works the other way too, if both legs are the same length (indicated by a line draw through both sides) the angles opposite to the same length legs are 45°.

In a 30°, 60°, 90° triangle, The hypotenuse is twice the length of the shortest side (the one opposite the 30° angle) and the other leg (the one opposite the 60° angle) is the short leg times
`√(3)`

• If a triangle has two angles out of 30°, 60°, 90°, the last angle must be the only other different angle (because they have to sum to 180)

1. Your answer to the first problem is correct.

2. Because there is a line through two of the sides, they are the same length, and the hypotenuse is
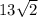`13√(2)`, the sides are both of length
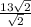`(13√(2))/(√(2))` or 13.

3. We can see that there is a 90° angle (from the square in the corner) and a 45° angle. This means that the two legs have equal length. One leg is
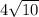`4√(10)` so the other is the same. The hypotenuse is
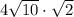`4√(10)  \cdot √(2)`, or
`8√(5)`

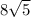
4. The hypotenuse is twice the length opposite the 30° angle (9), so it's length is 18. The last angle must be 60° and the side opposite the 60° must be
`9√(3)`

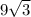
5. There are two 45, 45, 90 triangle that make up a square. The hypotenuse of both of these is 24, meaning the side lengths are
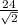`(24)/(√(2))`, or, (rationalized),
`12√(2)`

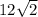
6. 30 60 90 triangle, short side is
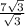`(7√(3))/(√(3))` or 7 and hypotenuse is twice that length, so it is 14.

7. 45 45 90 triangle, hypotenuse is 7 so lengths are
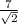`(7)/(√(2))` or
`(7√(2))/(2)`

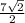
8. 30 60 90 triangle, longer leg is 18, shorter leg is
`6√(3)`, hypotenuse is
`12√(3)`,

9. 45 45 90 triangle, hypotenuse of
`3√(6)`, side lengths of
`3√(3)`

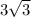
10. 30 60 90, hypotenuse of 28, shorter leg 14, longer leg
`14√(3)`

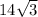
11. 30 60 90, hypotenuse 15, shorter side
`(15)/(2)`, longer leg
`(15√(3))/(2)`

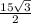
12. Two 30 60 90 triangles, hypotenuses of 16, shorter legs of 8 and longer legs of
`8√(3)`