Answer:
211.8 feet.
Explanation:
This problem models a right triangles, where the hypothenuses is the length of the string, and one leg would be 180ft, which is the difference between the total height from the ground and the pole height (186-6=180). Also, the angle in front of the knowing leg is 60.
As, based on all given values, we can apply trigonometric reasons to the hypothenuse, which is L.
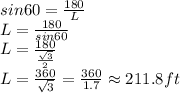
Therefore, the length of the kite string is around 211.8 feet.