Question

Find the equation of a line through these two points (9,11) (21,27)

Answer 1

1) The equation of the line in point slope form is equal to
`y-11=(4)/(3)(x-9)`

2) The equation of the line in slope intercept form is equal to
`y=(4)/(3)x-1`

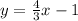
3) The equation of the line in standard form is equal to
`4x-3y=-3`

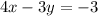
Explanation:

we have that the line passes through the points

(9,11) and (21,27)

step 1

Find the slope

The formula to calculate the slope between two points is equal to

`m=(y2-y1)/(x2-x1)`

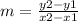
substitute the values

`m=(27-11)/(21-9)`

`m=(16)/(12)`

`m=(4)/(3)`

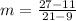
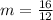
step 2

Find the equation of the line into point slope form

The equation of the line is

`y-y1=m(x-x1)`

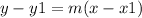
we have

`m=(4)/(3)`

`point (9,11)`

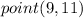
substitute

`y-11=(4)/(3)(x-9)` -----> equation of the line into point slope form

step 3

Find the equation of the line in slope intercept form

`y=mx+b`

`y-11=(4)/(3)(x-9)`

`y=(4)/(3)x-12+11`

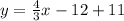
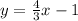`y=(4)/(3)x-1` -----> equation of the line in slope intercept form

step 4

Find the equation of the line in standard form

`Ax+By=C`

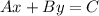
where

A is a positive integer

B and C are integers

`y=(4)/(3)x-1`

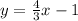
Multiply by 3 both sides to remove the fraction

`3y=4x-3`

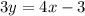
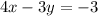`4x-3y=-3` ----> equation of the line in standard form