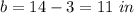Please Help! The base of a triangle is 3 inches shorter than its height. The area of the triangle is 77 square inches. Find the base and the height Explain Step-by-step please I…

Question

asked Sep 11, 2020 37.0k views

1 Answer

Destan · Answer 1 · 2020-09-17T05:16:40+0000

Answer:

The base is
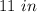$11\ in$ and the height is
$14\ in$

Explanation:

we know that

The area of the triangle is equal to

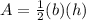
A=(1)/(2)(b)(h)

where

b is the base of triangle

h is the height of triangle

In this problem

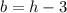b=h-3 ----> equation A

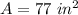
$A=77\ in^(2)$

so

77=(1)/(2)(b)(h)

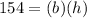154=(b)(h) -----> equation B

Substitute equation A in equation B

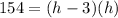
154=(h-3)(h)

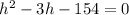
h^(2)-3h-154=0

Solve the quadratic equation by graphing

The solution is
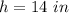
$h=14\ in$

see the attached figure

Find the base b

$b=14-3=11\ in$