Question

What are the x-intercepts of the graph of the equation above?

Answer 1

B. (-3, 0) and (-6,0)

Explanation:

x intercept when y = 0

so x^2 + 9x + 18 = 0

Factor

(x + 6)(x + 3) = 0

x+6 = 0; x = -6

x+3 = 0; x = -3

Answer

B. (-3, 0) and (-6,0)

Answer 2

Answer: Option B.

Explanation:

The graph of the quadratic equation is a parabola.

The parabola intercepts the x-axis when
`y=0`.

Then, you need to substitute
`y=0` into the equation:

`y=x^2+9x+18\\0=x^2+9x+18`

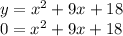
Factor the quadratic equation: find two number that when you add them you get 9 and when you multiply them you get 18. In this case these numbers are 6 and 3. Then:

`0=(x+6)(x+3)\\\\x_1=-6\\x_2=-3`

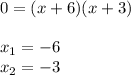
Therefore, the x-intercepts are:

(-3,0) and (-6,0)