Question

Help quick , I’m being timed

Answer 1

Hello!

The answer is:

Both
`x=-2` and
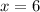`x=6` are true solutions.

Why?

We need to remember that an extraneous solution are not really a solution, it often happens when mathematic indetermination are formed, for example, a dividing a number by 0. On the opposite, a real solution is a solution is a real number.

We don't need to check for a extraneous solution since there is not any restriction to the given equations. Since we are working with cubic roots, there is not any restriction.

So,

Evaluating both "x" values, we have:

Evaluating,
`x=-2`

`\sqrt[3]{x^(2) -12} -\sqrt[3]{4x}\\ \\\sqrt[3]{(-2)^(2) -12} -\sqrt[3]{4*(-2)}\\\\\sqrt[3]{4 -12} -\sqrt[3]{-8}=\sqrt[3]{-8}-\sqrt[3]{-8}=-2-(-2)=0`

So, evaluating
`x=-2`, we know that it's a real solution.

Evaluating,
`x=6`

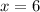
`\sqrt[3]{x^(2) -12} -\sqrt[3]{4x}\\ \\\sqrt[3]{(6)^(2) -12} -\sqrt[3]{4*(6)}\\\\\sqrt[3]{36 -12} -\sqrt[3]{24}=\sqrt[3]{24}-\sqrt[3]{24}=0`

So, evaluating
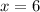`x=6`, we know that it's a real solution

Hence,

Both
`x=-2` and
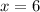`x=6` are true solutions.

Have a nice day!