Question

How do I solve this?

Answer 1

C. 100π

Explanation:

The formula of a volume of a sphere:

`V=(4)/(3)\pi R^3`

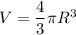
The formula of a surface area of a sphere:

`S.A.=4\pi R^2`

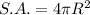
R - radius

We need the length of the radius to calculate the area of the sphere.

Calculate it from the volume of the sphere.

We have a volume:

`V=(500)/(3)\pi\ cm^3`

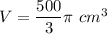
Substitute to the formula of a volume:

`(4)/(3)\pi R^3=(500)/(3)\pi R^3` divide both sides by π

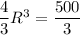`(4)/(3)R^3=(500)/(3)` multiply both sides by 3

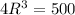`4R^3=500` divide both sides by 4

`R^3=125\to R=\sqrt[6]{125}\\\\R=5\ cm`

Put the value of radius to the formula of a surface area of a sphere:

`S.A.=4\pi(5^2)=4\pi(25)=100\pi\ cm^2`