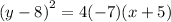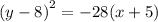ANSWER
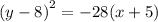
Step-by-step explanation
The equation of a parabola whose axis of symmetry is parallel to the x-axis is given by;
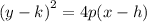
where (h ,k)=(-5,8) is the vertex and p is the focal length.
The focal length is the distance from the focus to the vertex.
This is equal to the distance from the vertex to the directrix.
p=2--5=7
But because the parabola opens in the negative direction of the x-axis, its equation becomes,